Questions and Answers about Multiplication Fact Research
The research is more limited than you might think.
I love writing about multiplication, but math facts research is a real pain.
This is a follow-up to the my addition facts post. The problem with addition facts is the complexity and size of its literature. The size of that project was at times overwhelming, and I felt strained by the need to bring it all together.
I was therefore surprised when I started looking for studies on multiplication facts. There’s much less here!
And don’t bother asking about subtraction or division. There’s even less for those operations. It’s a shame because the work of memorizing addition and multiplication seems really different from my classroom perspective, and I have a lot of questions about how best to teach it.
In light of the highly visible successes of the “science of reading” movement in challenging progressive educators, some have been looking for a mathematical equivalent. A group of researchers have even put forward a vision of what that could look like, but I think any such talk is largely aspirational. To see why, look no further than multiplication fact research, where the most significant questions and debates about teaching multiplication facts are all, in fact, unsettled.
Should mental strategies precede fact instruction? If so, which strategies? How important is skip-counting? What flashcard routines tend to work for typical students? Do some timed routines make students anxious?
I have some of my own answers to these questions, but research hasn’t touched many of them, making the teaching of multiplication something less than science, as far as I can tell. But let’s get to the research, and you can decide for yourself.
***
Q: How do students solve multiplication problems?
A: They either calculate them using some strategy, or they know them by heart.
Q: Do adults answer all single-digit multiplication problems by heart?
A: No, they calculate many of them.
In 2 studies, undergraduates described their solutions of single-digit multiplication problems. They reported direct retrieval on approximately 80% of trials, but also reported rules (e.g., anything times 0 is 0), repeated addition (e.g., 2X 4 = 4 + 4), number series (e.g., 3 X 5 = 5, 10, 15), and derived facts (e.g., 6 X 7 = [6 X 6] + 6).
Campbell, J. I., & Xue, Q. (2001). Cognitive arithmetic across cultures. Journal of experimental psychology: General, 130(2), 299.
The results indicate that procedural strategies constitute a major part of educated adults' repertoire of simple-arithmetic skills. Indeed, exclusive reliance on retrieval for simple arithmetic probably is a rare achievement.
Q: Given that even adults use computational strategies, does it even matter if students know multiplication facts by heart?
A: Yes, because knowing multiplication by heart—memorization—is helpful for learning other mathematical skills, especially fraction procedures. We know because even when you control for everything else, knowing how well a kid knows multiplication facts helps you know if they will struggle with fractions years later.
Hansen, N., Jordan, N. C., Fernandez, E., Siegler, R. S., Fuchs, L., Gersten, R., & Micklos, D. (2015). General and math-specific predictors of sixth-graders’ knowledge of fractions. Cognitive Development, 35, 34-49.
For fraction procedures, attention and whole number computational abilities (multiplication fluency and division) emerge as key predictors, as has been noted in previous research (e.g., Hecht & Vagi, 2010)… In contrast, multiplication fluency was uniquely predictive fraction procedures but not concepts, which makes sense given the importance of multiplication for finding common denominators (e.g., 3/4 + 2/3).
Q: Is this surprising?
A: No, because knowing a fact by heart essentially removes a step (“calculate the product”) from a multi-step procedure. We should also expect memorization to help with multi-digit multiplication or word problems, even without those big correlational studies.
Q: Would giving students a multiplication fact “cheat sheet” also improve the learning of these fraction procedures? Wouldn’t that also remove a step from that fraction procedure?
A: I think that makes sense, but there is no research on this.
Q: How do people memorize a fact?
A: By remembering the numbers in the problem (“3 x 7”) along with the product (“21”). That association is stored as part of a network of related facts.
Dunlosky, J., Rawson, K. A., Marsh, E. J., Nathan, M. J., & Willingham, D. T. (2013). Improving students’ learning with effective learning techniques: Promising directions from cognitive and educational psychology. Psychological Science in the public interest, 14(1), 4-58.
Attempting to retrieve target information involves a search of long-term memory that activates related information, and this activated information may then be encoded along with the retrieved target, forming an elaborated trace that affords multiple pathways to facilitate later access to that information.
Q: Is memorization hard because kids don’t understand the meaning of multiplication?
A: No, multiplication is typically understood well from an early age.
Sherin, B., & Fuson, K. (2005). Multiplication strategies and the appropriation of computational resources. Journal for research in mathematics education, 36(4), 347-395.
Furthermore, by the time of formal instruction, students already possess the fundamental conceptual capabilities required for conceptualizing multiplication. Indeed, it has been documented that, as early as kindergarten, children can solve simple multiplication problems.
Q: Do most students go through a stage where they use strategies before they memorize a fact?
A: Yes, it seems so.
Q: Is there a typical development of strategies used to answer multiplication questions?
A: Sort of! But it’s not like addition, where students learn strategies that they can apply to all addition problems. Multiplication involves much more number-specific knowledge, i.e. knowledge about specific numbers like 4, 12 and 32. This impacts the strategies that students use.
Sherin, B., & Fuson, K. (2005). Multiplication strategies and the appropriation of computational resources. Journal for research in mathematics education, 36(4), 347-395.
Stated simply, students acquire a great deal of kn about specific numbers-such as 4, 12, and 32-and this knowledge allows of new strategies or the use of old strategies in new contexts. For this reason the central issues associated with the learning of single-digit multiplication are very different from those associated with addition.
Q: Why does it impact the strategies students use?
A: The usefulness of a given computation strategy for multiplication varies a lot depending on the numbers involved and how much students know about them. As a consequence, students will tend to use a variety of mental strategies for different problems using e.g. skip-counting for 5s while also using doubling to compute 6 x 4 (since 6 + 6 = 12 is well-known) but not for 4 x 8 (because 16 + 16 is harder than 12 + 12).
Sherin, B., & Fuson, K. (2005). Multiplication strategies and the appropriation of computational resources. Journal for research in mathematics education, 36(4), 347-395.
This stance has significant implications for the manner in which we can to achieve our stated goals. Most dramatically, this stance moderates the extent to which we can expect to develop a simple and universal account of learning progressions in multiplication strategies. Strategy use by individuals, in a particular circumstance, will be very sensitive to the number-specific resources able, which are in turn sensitive to details of instruction.
Q: Fine, but what are those strategies that students typically use?
A: Students usually start by representing groups, like maybe drawing a picture of 4 x 5 but counting each of the 20 elements one-by-one (“count all”). Then students move to some sort of laborious repeated addition. Then to skip-counting. Students often learn some rules for x1, x0, x10, and then there is memorization and using memorized facts to derive new ones.
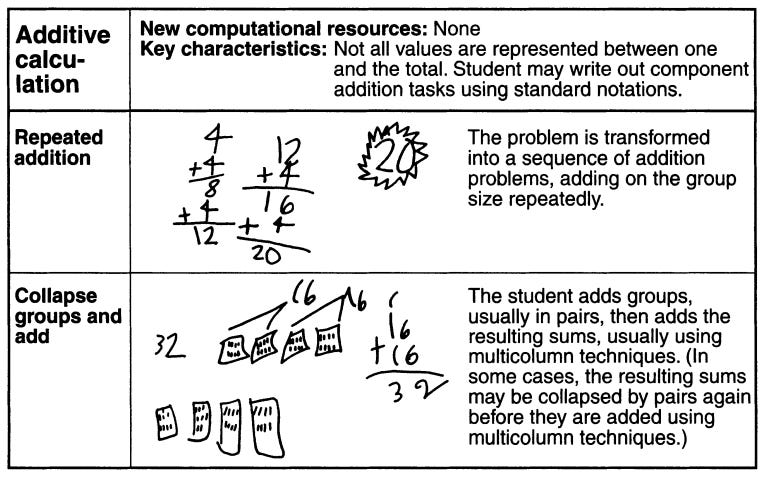
Q: There is evidence that you can improve kids’ addition fact knowledge by explicitly teaching the “counting on” strategy. But this is hugely contentious in multiplication, where some educators claim that students should learn strategies such as “decomposition” or “doubling/halving” and others say that students should go straight to memorization. Who’s right?
A: There is precisely one study I know of that has considered this question. That’s it.
The study found that strategy instruction helped a bit, which is all well and good, but you probably don’t want to make generalizations from any one paper, especially one involving not that many students and using curricular materials designed by the researchers.
Woodward, J. (2006). Developing automaticity in multiplication facts: Integrating strategy instruction with timed practice drills. Learning Disability Quarterly, 29(4), 269-289.
Q: So, we don’t know if instruction in strategies helps memorization?
A: No, not really. And for whatever reason, the issue has barely been studied.
Q: Why wouldn’t using strategies lead to automaticity?
A: Think about skip-counting, a common multiplication strategy. We memorize 6 x 7 = 42 by explicitly thinking about the numbers 6, 7, and 42 together in a multiplication context (“rehearsal”).
If you skip-count to solve 6 x 7, you will list 7, 14, 21, 28, 35, 42. First, there are many opportunities for error. Second, a student might not be thinking about 6 x 7 by the time they reach the answer—they may be thinking mostly about 35 + 7, and no connection is made. (I wrote more about this here.)
Q: What about more efficient strategies? Conceivably, thinking about (7 x 5) + 7 would give a student some chances to think about 7, 6 and 42 in tandem, even if skip-counting wouldn’t?
A: There is no research about this that I know of. But it seems to me that it depends on how automatic a student is with 7 x 5, and it may very well be this kind of strategy allows students to make the “7, 6, & 42” connection. In this way, it could be that students with automaticity in some facts can use efficient strategies to become automatic in more. But, again, there is no research on this at all that I can find.
Q: What do you think?
A: I think teachers should first have students memorize smaller facts, then teach more efficient strategies using those facts, then have students memorize the larger facts. That way the efficient facts are actually efficient, and we can talk about the properties of multiplication that generalize to multi-digit problems anyway.
Q: Do students with learning disabilities struggle with multiplication facts?
A: Yup.
Campbell, J. I., & Graham, D. J Psychology. (1985). Mental multiplication skill: Structure, process, and acquisition. Canadian Journal of /Revue canadienne de psychologie, 39(2), 338.
Research on primary grade students indicates that students with LD are more likely to rely on counting strategies than direct retrieval when working single-digit fact problems (Geary & Brown, 1991; Hanich, Jordan, Kaplan, & Dick, 2001; Hoard, Geary, & Hamson, 1999)
Q: Are there routines that help students remember multiplication facts?
A: There are a variety of routines. They have mostly been developed to help students with learning disabilities memorize math facts. And they all share two main features:
Students are presented with an unknown fact
Students are asked to practice it with a time-limit
The details of the routines vary. For example, in “taped problems” a recording (I guess it could be a teacher) reads the problems aloud, then pauses, then says the product. Students are asked to “beat the tape” as often as they can, but otherwise to write what the tape says.
McCallum, E., Skinner, C. H., Turner, H., & Saecker, L. (2006). The taped-problems intervention: Increasing multiplication fact fluency using a low-tech, classwide, time-delay intervention. School Psychology Review, 35(3), 419-434.
You will be listening to a tape with multiplication problems and answers. Follow along on your sheet and try to write the answer to each problem before it is spoken on the tape. If you write a wrong answer, mark through it with a slash and write the correct answer as you hear it on the tape. If an answer is given before you can come up with it on your own, write the correct answer as it is said on the tape. Try your best to beat the tape but do not skip ahead. When the tape is over, I will collect the sheets. Ready? Begin.
Another routine is “cover, copy, compare,” and in it students read a few equations, cover them up, try to write them from memory, and then compare their answers to the original covered problems.
Poncy, B. C., Fontenelle, S. F., & Skinner, C. H. (2013). Using detect, practice, and repair (DPR) to differentiate and individualize math fact instruction in a class-wide setting. Journal of Behavioral Education, 22, 211-228.
CCC takes the learner through 5 steps: (a) the student will review the target mathematic problem, (b) study the mathematic problem with the answer, (c) cover the problem and answer, (d) write the problem and answer, (e) uncover the original problem and answer and compare their response to the original printed problem and answer.
Q: Why is there a time-limit?
A: To make sure that students are using their memories and not using a computational strategy.
McCallum, E., Skinner, C. H., Turner, H., & Saecker, L. (2006). The taped-problems intervention: Increasing multiplication fact fluency using a low-tech, classwide, time-delay intervention. School Psychology Review, 35(3), 419-434.
Many students use time-consuming counting strategies to solve basic math facts (McCallum et al., 2004; Poncy, Skinner, & O’Mara, 2006). For example, when presented with the problem 2 x 9 , students are sometimes told to count by 2 for 9 units (e.g., 2, 4, 6, 8, 10, 12, 14, 16, 18). Although such procedures may allow students to arrive at correct answers and promote a conceptual understanding of basic math operations, they may also prevent students from developing automaticity.
Q: Does the time limit cause math anxiety?
A: We don’t know, but this comes up often when people reflect back on their math schooling. And removing time limits seems to help alleviate the effects of math anxiety.
Harper, N. W., & Daane, C. J. (1998). Causes and reduction of math anxiety in preservice elementary teachers. Action in Teacher Education, 19(4), 29-38.
The study showed that there was a significant reduction in the level of math anxiety at the end of the methods course. Factors causing the original anxiety centered around an emphasis on right answers, word problems, fear of making mistakes, timed tests, and confidence levels.
Chinn, S. (2009). Mathematics anxiety in secondary students in England. Dyslexia, 15(1), 61-68.
Over 2000 students in independent and mainstream schools in England completed a 20-item questionnaire designed to investigate maths anxiety levels. The same questionnaire was given to over 440 dyslexic males in specialist schools within the same age range. The results showed that examinations and tests create high levels of anxiety in approximately 4% of students.
Ashcraft, M. H., & Krause, J. A. (2007). Working memory, math performance, and math anxiety. Psychonomic bulletin & review, 14, 243-248.
Likewise, when we gave untimed paper-and-pencil tests of our whole-number arithmetic stimuli, we found no math-anxiety differences on accuracy, even though these same stimuli generated online anxiety effects in an RT task.
Q: What do you think is going on?
A: I think timed memorization activities that accidentally prompt students to use computational strategies can actually cause math anxiety, and it’s best to either use flexible timing or figure out ways to make sure students aren’t using strategies. And, though there’s no evidence for this, I suspect the abuse of “Mad Minute” activities is to blame. (There’s plenty of instances of these types of timed “probes” being used in OK ways—they’re all over the special education interventions literature.)
Q: What about flashcard routines?
A: Incremental rehearsal is a flashcard practice strategy that has students practice a single unknown card at longer and longer intervals by inserting cards with known facts into the mix. Naturally, it takes longer, but it has often been found to lead to better retention than a big pile of unknown facts for struggling students. The lesson here, I believe, is to move systematically in managable bites with fact practice.
Burns, M. K., Aguilar, L. N., Young, H., Preast, J. L., Taylor, C. N., & Walsh, A. D. (2019). Comparing the effects of incremental rehearsal and traditional drill on retention of mathematics facts and predicting the effects with memory. School Psychology, 34(5), 521.
Q: How well do these routines work in a full classroom with typical students, instead of students with learning disabilities in small settings?
A: There are a few studies here and there, but basically, there isn’t much research on this. If you want a small study in a class-wide setting, here is a nice one:
Poncy, B. C., Fontenelle, S. F., & Skinner, C. H. (2013). Using detect, practice, and repair (DPR) to differentiate and individualize math fact instruction in a class-wide setting. Journal of Behavioral Education, 22, 211-228.
(I have my own routine that I think works pretty well.)
Q: Do researchers know which facts students find the hardest to memorize?
A: Yes.
Q: How?
A: By measuring how long it takes for people to answer a multiplication question, and by keeping track of the errors that they make. There are a handful of things that can make a problem easier to memorize:
Problem-size effect – All things being equal, smaller facts are easier to memorize.
Tie effect – 6 x 6 is easier than 5 x 7, probably because there are fewer digits to coordinate.
Order effect – 9 x 2 is often easier than 2 x 9 (because students think of it as 2 nines instead of 9 twos)
Operand effect – There are nice patterns for facts involving 0, 1, 2, 5, and 9.
van der Ven, S. H., Straatemeier, M., Jansen, B. R., Klinkenberg, S., & van der Maas, H. L. (2015). Learning multiplication: An integrated analysis of the multiplication ability of primary school children and the difficulty of single digit and multidigit multiplication problems. Learning and Individual Differences, 43, 48-62.
Differences exist not only at the level of the child, but also at the level of math problems; not all single digit multiplication problems are solved equally fast and accurately, even by adults. For example, 8 × 7 is more difficult than 2 × 2. Different effects have been identified in the literature, explaining systematic differences in response time and accuracy between problems: the problem size effect, the tie effect, the order effect, the parity effect and effects related to specific operands.
Q: What are the educational implications of this?
A: I don’t know. Maybe to teach the easy facts first?
Q: So you’re saying that we have evidence that multiplication memorization is important. We know, more or less, how memorization happens when isolated, but we don’t actually know if strategy instruction helps with any of that. And there is no strategy that we know of that is actually “crucial,” it’s more about students learning a lot about a lot of different numbers. (This is different from addition!) And while there are memorization routines that have been developed for struggling students in small settings, there isn’t really a “consensus” way to promote memorization in a classroom. Those routines would have to be adopted for your setting. Basically, what we have are principles—make sure kids have a chance to rehearse a fact before practicing it, don’t try to memorize too much at once, use time limits to short-circuit attempts to encourage kids to use their memories to answer, but don’t use time limits in a way that stresses kids out, give quick feedback and practice a lot. Right?
A: Right.
Q: Any last words?
A: Oh yeah, mnemonics don’t work as well as rehearsal practice.
Nelson, P. M., Burns, M. K., Kanive, R., & Ysseldyke, J. E. (2013). Comparison of a math fact rehearsal and a mnemonic strategy approach for improving math fact fluency. Journal of school psychology, 51(6), 659-667.
Students in the practice-based intervention group had higher retention scores.
I don’t really like mnemonics.
Thanks for reading! I’m a classroom mathematics teacher and a writer. If you like what you read, you might also like my book Teaching Math With Examples.
And if you liked this post, please consider sharing it on social media and the like. It helps me get more subscribers, sell more books, put kids in summer camp, buy new pants, etc.