Colin Roberts claims to have discovered the sphericon in 1969. I don’t doubt that he thought it up, but was he really the first? The sphericon is such a natural shape—a double cone, split from tip-to-tip, then twisted—that I’d be shocked if Roberts was the first guy in history to stumble across it.
But then again, who am I to say? I didn’t come up with it. Fine—Colin Roberts discovered the sphericon in 1969, and here’s the cool thing about it: it wobbles.
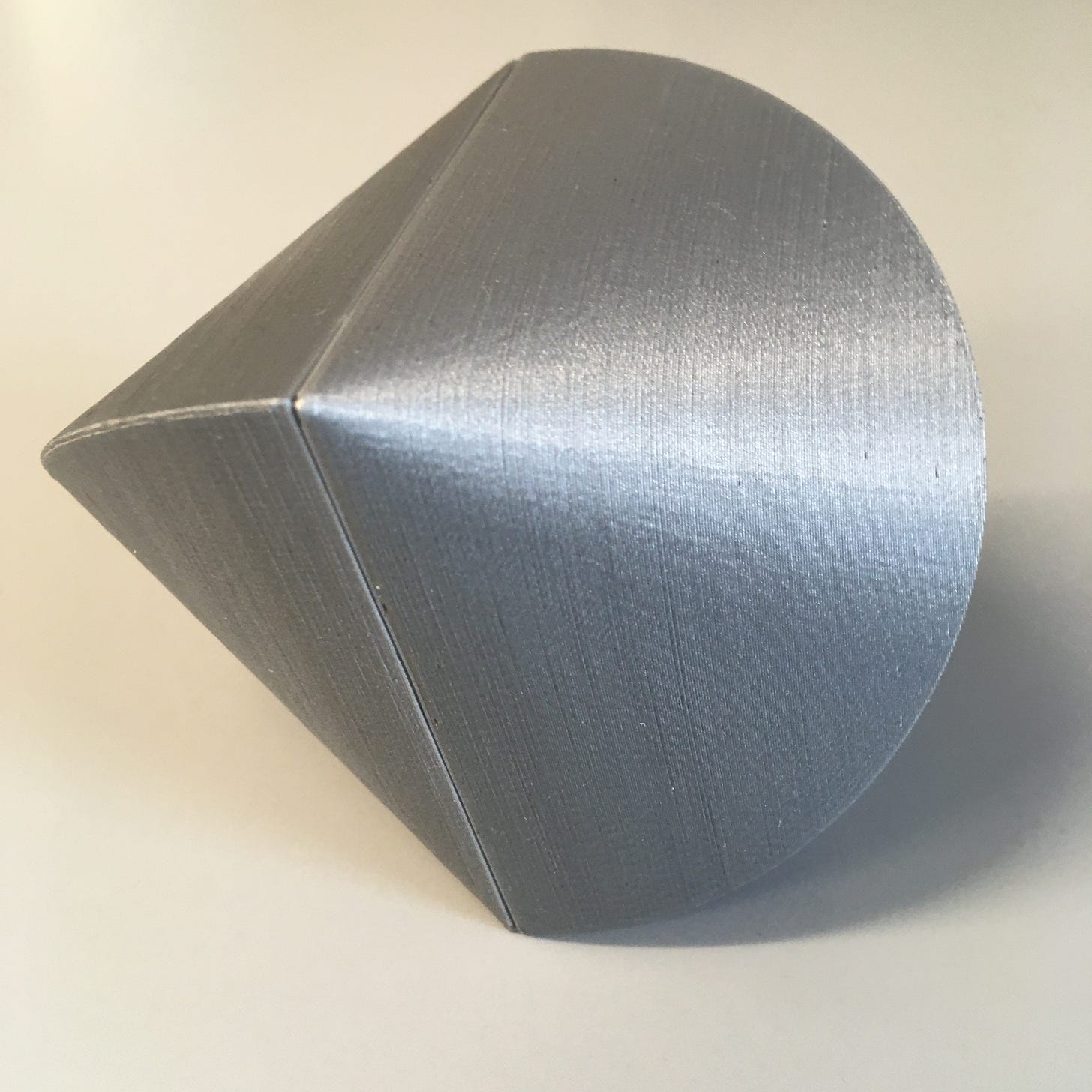
I begged my brilliant colleague to use the school’s 3D printer to print me one, and he kindly provided me with this:
It’s made of two pieces, each one half of a bicone. You can see this more clearly if you twist those pieces, which sends you right back to the bicone.
If you’re looking straight on at the sphericon (as in the above image) you can sort of see that it has square dimensions. It does! And the angle from the apex extending down makes a 90 degree angle.
(I was trying to imagine if you could have one of these things with a different angle, but it wouldn’t work—if the angle were smaller, it would be taller than its radius and the whole thing wouldn’t fit. It needs to be square.)
Paula Krieg is a woderful friend, and she has a wonderful blog post where she shares templates for folding up your own vibrantly colorful sphericons. Those templates, incidentally, also show you the path that the sphericon takes as it rolls. It happily rolls along on one cone, then smoothly switches to the other, rolling (temporarily) in the opposite direction. But then it rights its path, and eventually wobbles along in a straight(-ish) line.

My class is currently studying volume and surface area, so as a little challenge assignment I asked them to find the volume of the sphericon. Not so bad, as far as these things go! The volume is equal to that of the bicone, and the height of each of those cones is equal to the radius (because of the square thing). Using the cone volume formula, its volume is:
Its surface area is just double that of each cone’s, and again the height is equal to the radius:
Eric Weissstein’s MathWorld doesn’t usually editorialize, but on the sphericon he can’t help himself. “This solid is not as widely known as it should be,” he writes on his entry on the sphericon. I agree, but also I get it—the sphericon is a one-trick pony. Granted, it’s a delightful and surprising trick, but like a lot of cool things I’m left with a feeling of, OK, so what do we do we this?
One thing we—people, mathematicians, artists, etc.—do is generalize, and there are generalizations of the sphericon. David Hirsch and Katherine A. Seaton call them polycons, and they all roll in that great wobbly way. I don’t quite understand yet how they are constructed, but it’s all described in their paper (along with volumes and surface area formulas), and I’m still taking a shot at understanding it.
Update: Here’s how to construct them!
Anyway, there are hexacons, octacons, decacons, and if you click those links you can see them roll, and they look very pretty, I’m sure you’ll agree.
Generalization is one answer to the “what do I do with this” question. But there is another approach. Instead of abstraction, it moves in the other direction—adoration, specification. It says, what can we do with this sphericon?
It asks questions such as: could we make a sphericon? And, if so, could we make an absolutely GINORMOUS sphericon? And couldn’t we put a person in it? Sure we could! And would it be fun to see a person wobbling along inside it?
How lucky we are that MOMIX (a dance company) has answered all these questions in the affirmative, with a piece titled “Circle Walker.” I showed a few minutes of it to my students, it’s absolutely stunning.
And that’s all I know about the sphericon, this week’s shape of the week.
Stray Links:
There is a connection between the a different generalization of the sphericon, the octasphericon, and mazes, according to this page.
This piece by Henry Segerman about rolling acrobatic apparatuses (apparati?!) goes into depth about…something, I didn’t actually read it, but I see sphericons all over the place.